A blog from Tim Worstall at the Adam Smith Institute claims bizarrely that the way to cut unemployment is to cut unemployment benefits. That’s like blaming hospitals for broken legs – though I suppose that if there weren’t as many hospitals we’d not get to count the broken legs quite so thoroughly.
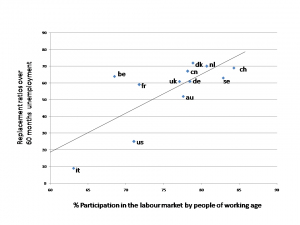
I threw together this graph fairly rapidly from OECD figures I had to hand: the axis on the left shows replacement ratios (how much unemployment benefits replace of salary during the first 60 months, assuming housing costs are allowed for), the bottom axis shows labour market participation, the points on the graph are all major OECD countries. If there is a relationship, it’s in the opposite direction to the claim made by the Adam Smith Institute. I’m sceptical, however, that there is a direct relationship. There is no good theoretical reason to suppose that unemployment benefits trump economic and social conditions in determining labour market participation.
So you didn’t even bother to discuss the specific data that I mentioned?
Well done, well done. Especially as the point was much more about the length of time that benefits were paid for, not the amount of them….
You’ll see that the OECD statistics I used for replacement ratios (http://www.oecd.org/els/benefits-and-wages-statistics.htm) are based on duration over 60 months. The case you cite comes from Florida, and that simply can’t support the generalisation you make. (Nor, because of the common economic and policy framework, would further data from the US – you’d also have to show that variations between states were not due to local economic conditions, which it’s fiendishly difficult to do.) Some countries, such as Italy and France, offer replacement ratios that get reduced over time, without apparently improving unemployment rates. However, counter evidence from those states would fail just as badly to support any generalisation as your example does.
What I have done is to link to your argument and draw attention to it. In respect of my own comment, I’ve posted some of my own reservations about this sort of data.